Mathematical analysis of the kolam patterns
The kolam patterns used in India are not new.... they have been there for aeons, have ancient origins and were made with the purpose of propitiating Gods, and Goddesses and were celebratory in nature. They were a form of floor art or paintings, made with devotion. Many of these were derived from purely secular motifs; the abstract designs, philosophic and religious motifs mingled together and these symbols expressed the feelings of people and were highly aesthetic too. Their presence during all the various festive occasions reinforced the importance and the spirit of the occasion.
These forms of painted prayers were not only handed down to the generations with their core wisdom intact but have been enhanced by each generation, be it in terms of the materials or patterns used, but also by the technology present in that era. The traditionalists still follow the age-old methods of using rice powder or creating the patterns with dots, at the thresholds of homes and courtyards but in this day one sees the presence of plastic rangoli or kolam stickers, plastic rangoli templates, plastic rangoli rollers, plastic grid sheets which help to create an instant and accurate rangoli grid as an aid to creating the rangolis.
As rangolis are more predominant in rural areas than in urban areas, it is necessary to appreciate the ease with which these designs are passed down from one generation to the other, by mere daily practice and retention in the memory. Though in the past few years, there have been dedicated rangoli pattern books; a few publications have a section which prints the rangoli explorations of the enthusiasts of this art form.
All the different types of rangolis, across the country exhibit a sense of geometry, and follow some basic rules. Rangolis are not random patterns; they all follow an underlying structure and the vast collection of designs of all types indicates that a certain discipline governs either the simplicity or the complexity of the pattern.
Different sets of rules have to be followed for generating a particular type of rangoli. Also the patterns range between geometric and mathematical line drawings around a matrix of dots to free-form artwork and closed shapes.
Generally to generate the kolams, dots are drawn first on the floor and then depending on the type of kolam, lines are drawn either connecting the dots or going around the dots and complete as closed curves. The patterns where the lines are drawn around the dots are called as Kambi kolams as they look like wire decoration (Kambi means “wire” in Tamil). There are many interesting and complicated designs made up of single or many closed curves.
Constrained only by some very broad rules, kolam designs offer scope for intricacy, complexity and creativity of high order. These traditional designs also seem to imbibe mathematical properties such as symmetry, permutation etc Rangoli patterns have evoked a sense of awe in all.....as they are visual symbolic expressions. The presence of geometry and sometimes symmetry has led some of thinkers, and mathematicians to study them. They are a cultural and artistic expression of a whole array of mathematical ideas and concepts.
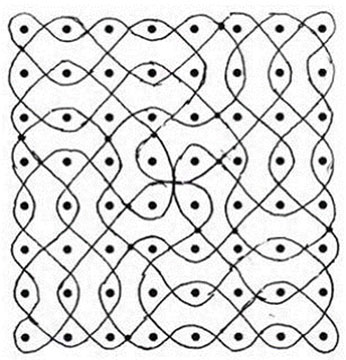
In the past few years a few mathematicians, physicists have explored these patterns, to see if any standard theories apply to the generation of rangolis. Because of the basic grid structure of dots used in the kolam type of rangoli, computational analysis was explored. Kolams come in a variety of styles (which the computer scientists have taken to calling “kolam families”). Since many begin with a grid of dots, this serves as the skeletal structure for the kolam. Lines are drawn, connecting the dots or around them, very often in a single, continuous curve. (This continuous curve is supposedly symbolic of the never-ending cycle of birth, fertility, death, continuity and eternity). The finished product is a symmetrical kolam.
Kolams come in a variety of symmetries – horizontal, vertical and rotational.
(Image source)
The fascination the humble kolams have generated is seen in the technical explorations using the computers and algorithms, to arrive at a new scientific understanding. Trying to generate them on a computer, creation of new models has brought in an entirely new dimension in the otherwise non-technical floor art.
Theories like the Fibonacci numbers have been applied by retired Dr. Naranan to kolams to arrive at interesting conclusions. (Fibonacci numbers are pervasive in all areas of science. In geometry, they are related to pentagons, decagons and 3-dimensional Platonic solids. Rectangles of consecutive Fibonacci numbers as sides (e.g. 2 x 3, 3 x 5, 5 x 8, 8 x 13...) are known as Golden rectangles. The ratio of the sides approaches a limiting value denoted by D = (1+E5)/2 = 1.61803.... is called the Golden Ratio. This ratio is everywhere in nature: in the branching of trees, arrangement of flower petals, seeds and leaves, spiral patterns of florets in sunflowers, spiral shapes of sea shells etc. It appears to be closely related to growth processes in nature. The Golden Ratio is also believed to figure prominently in Western art: in architecture (pyramids, Parthenon), paintings (Leonardo da Vinci), sculpture, poetry (Virgil) and music. There is a vast literature on this subject and many claims are controversial.
The Fibonacci series first appeared in the book Liber Abaci (1202) by the Italian mathematician Leonardo of Pisa also known as Fibonacci. (It is claimed that these numbers appeared in the analysis of Sanskrit poetry by Acharya Hemachandra in 1150 A.D, 50 years before Liber Abaci.) Dr. Naranan has explained how kolam designs, square and rectangular, can be constructed using a modular approach. Bigger kolams are built from smaller ones using some properties of Fibonacci numbers.
The modular approach is ideally suited for generating kolams on a computer and exploring interesting mathematical properties of kolams in general. He has incorporated Fibonacci numbers in a class of artistic designs he calls Fibonacci kolams (FKs). He has also developed a recursive scheme to generate Fibonacci rectangles and squares using algorithms that can be used to draw FKs on a computer with suitable software.
He believes that computer-aided kolam design will elevate art to a new level of creativity. A computer program becomes indispensable if one has to explore the different possible combinations of rectangular and square modules that constitute the kolam.
He has presented his study and analysis in two parts. Part I consists of a scheme for creating a class of kolams based on Fibonacci numbers. In Part II, the scheme is generalized to arbitrary sizes of kolams using Generalized Fibonacci numbers. The problem of enumeration – the number of possible Fibonacci kolams of a given size – is discussed. This scheme is further extended beyond square grids to cover diamond-shaped grids too.
(Image source)
The ethnomathematics view and the corresponding study of kolam patterns have been done extensively by others like Ascher (1991) and Gerdes (1989). T. Robinson’s studies have produced computer programs for the mechanical or interactive generation of kolam patterns. Studies also have been conducted by Nagata and Yanagisawa (2004) by the use of other underlying syntax rules and a digital expression for representing Kambi kolam patterns. Nagata and Robinson (2006) have extended the applicability of kolam designs as tangible pictures for people with disability. These are a few studies to indicate the explorations done on kolams.
Kolams have symmetry, patterned repetition, closed continuous curves and curve families, all of which have applications and meaning in mathematics and computer science. With their impeccably logical building up of patterns – their algorithmic nature - they have attracted the attention of computer scientists who have used kolams to study picture languages. Dr. Gift Siromoney of Madras Christian College, who was a leader in the study of the mathematical properties of kolams along with his team, has done some fascinating work on these kolams.
He was a pioneer in the study of kolam designs as part of the analysis and understanding of picture languages. The study of picture languages is closely related to formal language theory, which has been used by computer scientists in the study and development of programming languages. Picture languages use sets of basic units, and study the syntactic rules for combining these units. Using the rules of syntax for a specific kolam family, a computer can mechanically generate new kolam patterns. Kambi kolam patterns, fractals provided interesting examples of cycle languages. Kolam designs as examples of two-dimensional picture languages with formally definable syntactic rules, the formal properties of such languages have been studied by Dr. Gift Siromoney in 1972 and his group of scientists and mathematicians. They used these picture languages to describe and create kolam families. They created a language that produced strings of symbols, which could then be translated into pictures or kolams.
Array languages are another technique used for the mathematical and computational study of kolams, particularly those using dots. Motivated by the kolam designs, Dr. Siromoney and his team in 1974 have introduced different types of array grammars generating array languages and also have given specific instructions for drawing certain kinds of kolam patterns using Turtle motions with chain-code interpretation and kolam motions with cycle rewriting rules. These include Siromoney Matrix Grammars and Kolam Array Grammars. These arrays can be used to describe and create kolams of various shapes.
The studies done by Dr. Siromoney and Dr. Naranan in kolams educate one tremendously. They reinforce the spirit of exploration in man. They drive home the importance of one’s culture, and one is motivated to study it, understand it and innovate it thus preserving it for the future generations. The complete technical details of their work and the work by some others in the area of kolams can be accessed through the following links.
These studies reinforce the idea that there is so much to learn about and from kolams. They also mean a lot more than just pretty patterns. They reflect the depth in our culture, connect us to our roots, urge us to see them in new light, and derive new meanings and understandings.

